Quantum Statistical Mechanics

QMHO

$\hat{H} = \frac{{\hat{p}}^{2}}{2 m} + \frac{m ω^{2} x^{2}}{2} = ℏ ω (b^{†} b + 1 / 2)$ with energies $E_{n} = (n + \frac{1}{2}) ℏ ω$ .

$Z_{Q H O} = \sum_{n} \exp (- β E_{n})$ .

$⟨ E ⟩_{Q H O} = ℏ ω (\frac{1}{2} + \frac{1}{\exp (β ℏ ω) - 1})$ with $⟨ n ⟩ = \frac{1}{\exp (β ℏ ω) - 1}$ - which looks like $⟨ n ⟩_{B E}$ with $μ = 0$ . BE is Bose-Einstein, $E (k) = \frac{ℏ^{2} k^{2}}{2 m}$ . We set $μ = 0$ since we can add or remove particles freely to this bosonic state without affecting the other particles.

Consider if we have an energy of $ℏ ω$ and we populate it with $n$ particles, then we get the same $\hat{H} = ℏ ω (b^{†} b + 1 / 2) = ℏ ω (n + 1 / 2)$ . Thus, we can consider a $n -$ energy level a bosonic gas of $n$ particles with energy $ℏ ω$ .

Maxwell Boltazmann Quantum Statistics

$Z_{N}^{M B} = \frac{1}{N!} Z_{N}^{d i s t i n g u i s h a b l e}$

$Z_{1} = \sum_{k} \exp (- β E_{k})$ . $Z_{N}^{N o n - I n t e r a c g i n t M B} = \frac{1}{N!} Z_{1}^{N}$ . Let $k = 1, 2, 3$ . $Z_{2}^{N I, M B} = \frac{1}{2!} {(\exp (- β E_{1}) + \exp (- β E_{2}) + \exp (- β E_{3}))}^{2} = \frac{1}{2} (\exp (- 2 β E_{1}) + \exp (- 2 β E_{2}) + \exp (- 2 β E_{3})) + \exp (- β (E_{1} + E_{2})) + \exp (- β (E_{2} + E_{3})) + \exp (- β (E_{1} + E_{3}))$ .

To fix partial occupancies, can take $\frac{1}{2} \to 1$ then $Z_{2}^{N I B o s o n s}$ or take $\frac{1}{2} \to 0$ then $Z_{2}^{N I F e r m i o n s}$ .

$Z_{G}^{N I, M B} = \sum_{M} Z_{M}^{N I, M B} \exp (M B μ) = \sum_{M} \frac{1}{M!} {(\sum_{k} \exp (- β E_{k}))}^{M} (\exp (β μ))^{M} = \sum_{M} \frac{1}{M!} {(\sum_{k} \exp (- β (E_{k} - μ)))}^{M} = \exp (\sum_{k} \exp (- β (E_{k} - μ))) = \prod_{k} \exp (- β (E_{k} - μ))$ .

$ϕ^{N I, M B} = - k T \ln Z_{G}^{N I, M B} = \sum_{k} ϕ_{k}$ . $ϕ_{k} = - k_{B} T \exp (- β (E_{k} - μ))$ , $⟨ n ⟩_{M B} = - \frac{\partial ϕ_{k}}{\partial μ} = \exp (- β (E_{k} - μ))$ .

So, $⟨ n ⟩ = \frac{1}{\exp (β (E - μ)) + γ}, γ = {\begin{cases} + 1 & F D \\ - 1 & B E \\ 0 & M B \end{cases}$ .

Plotting Behavior of E-mu

So, for MB this looks like a decaying exponential when plotted as a function of $E - μ$ . For BE, there is asymototic behavior at 0 but decays above the MB exponential as $E - μ$ increases. For FD, there it is half at zero and decays strictly below the MB exponeitial, at $- \infty$ it goes to 1.

At the classical limit, these different statistics converge to each other.

For very small $T$ , the BE curve approaches a delta function at $0$ . Since BE bounds the others above, the others become zero across the positive domain. The FD curve becomes a step-down function from $1$ to $0$ .

Plotting Behavior of E

The plots are shifted over by $μ$ . It gets more complicated if $μ$ is a function of temperature. Thus, the plots shift a bit left of $E = μ$ for a fixed value of $μ$ .

Classical Limits

$⟨ O ⟩ = \sum_{α} p_{α} O_{α}, p_{α} = \frac{1}{Z} \exp (- β E_{α})$ .

Quantum: $⟨ O ⟩ = \sum_{k} n (E_{k} - μ) O_{k}$ . $N = ⟨ N ⟩ = \sum_{k} n (E_{k} - μ) \to N (μ) \to μ$ .

For Fermions at $T = 0$ , $N = \sum_{k} 1 \to E_{k}^{m a x, o c c u p i e d} = μ \equiv E_{F} = ϵ_{F}$ , the Fermi energy.

Einstein Gas: basic energy is $ε = ℏ ω$ the constant, and the linear model is $ε = ℏ γ k$ . These give the optical phonons which are the approximately the constant and the acoustic are approximately the linear.

$ε (k) = \frac{ℏ^{2} k^{2}}{2 m}$ .

New Term

Review

BE and FD statistics
$⟨ n ⟩_{B / F} = \frac{1}{\exp (β (ϵ - μ)) \mp 1}$ . Recall, if $ϵ = μ$ then $⟨ n ⟩_{F} = \frac{1}{2}$ and $⟨ n ⟩_{B} \to \infty$ .

Classical: $⟨ E ⟩ = \sum_{α} p_{α} E_{α}$ , $p_{α} = \frac{\exp (- β E_{α})}{Z}$ . For a classical system, $Z = \frac{1}{N!} Z_{1}^{N}$ . For a quantum system, we can compute $⟨ E_{1} ⟩$ to then get $⟨ E ⟩ = N ⟨ E_{1} ⟩$ . I.e. for a carbon atom, since the electrons are dependent we can describe it in the classical way, but if they were independent then we would need FD or BE.

$⟨ E ⟩ = \sum_{\vec{k}} = n (ϵ (\vec{k}), T, μ (T)) ϵ (\vec{k})$ . Need: $μ (T)$ . For $N$ quantum particles, we have $N = N (μ) = \sum_{α} n_{α} = \sum_{\vec{k}} n (ϵ (\vec{k}), T, μ (T))$ . We can then invert this to get $μ (T)$ .

QHO

We can solve this classically since we have just one particle (i.e. one oscillator). $E_{n} = (n + \frac{1}{2}) ℏ ω$ . $⟨ E ⟩ = - \frac{\partial \ln Z}{\partial β}$ . $Z = \sum_{n} \exp (- β E_{n}) = \exp (- β ℏ ω / 2) \frac{1}{1 - \exp (- β ℏ ω)}$ . So, $⟨ E ⟩ = ℏ ω (\frac{1}{2} + \frac{1}{\exp (β ℏ ω) - 1}) = (⟨ n ⟩ + \frac{1}{2}) ℏ ω$ .

Density of States

$N (, μ, T) = \sum_{k} ⟨ n (ϵ_{k}, μ, T) ⟩ δ (ϵ - ϵ_{k}) = \int n (ϵ (\vec{k}), μ, T) g (ϵ) d ϵ$ .

Particles in a Box

$V = L^{D}$ , for $D -$ dimensions.

You can do this with:

Standing Waves

$Ψ \propto \sin (\frac{π}{L} n_{x} x)$ , $n \in Z^{+}$ . For the dimensions we have, each state takes up a volume of ${(\frac{L}{π})}^{D}$ . Note, we only integrate over one quadrant, hence $1 / 2^{D}$ of the total space $\to {(\frac{L}{2 π})}^{D}$ .

Plane Waves (Periodic Boundary Conditions)

So, $Ψ = \frac{1}{\sqrt{V}} \exp (i \vec{k} \cdot \vec{r})$ . Our $k$ is then, $\frac{2 π}{L} (n_{x}, n_{y}, n_{z})^{T}$ . Then, $n_{i} \in Z = {0, \pm 1, \pm 2, \dots}$ . For the dimensions we have, each state takes up a volume of ${(\frac{L}{2 π})}^{D}$ .

We need $ϵ (\vec{k})$ .

For massive fermions, $ϵ (\vec{k}) = \frac{ℏ^{2} k^{2}}{2 m}$ , from solving the Scrodinger Equation.
For massive bosons, $ϵ (\vec{k}) = c \cdot \vec{p} = c ℏ | \vec{k} | = c ℏ k$ .

If $ϵ (\vec{k}) \propto f (| \vec{k} |)$ . $g (ϵ) d ϵ = 4 π k^{2} \sum_{k_{i}} {| \frac{d k_{i}}{d ϵ} |}^{2} d ϵ \frac{V}{(2 π)^{3}} g (k)$ .

We use the simpler form, $g (ϵ) d ϵ = 4 π k^{2} {| \frac{d k}{d ϵ} |}^{2} d ϵ \frac{V}{(2 π)^{3}} g (k)$ For (1), $k^{2} = \frac{2 m ϵ}{ℏ^{2}}$ , $\frac{d k}{d ϵ} = \frac{1}{2} \sqrt{\frac{2 m}{ℏ^{2} ϵ}}$ . Then, $g (ϵ) d ϵ = \frac{V}{4 π^{2}} {(\frac{2 m}{ℏ^{2}})}^{3 / 2} \sqrt{ϵ} d ϵ$ .

Note, we could also write, $g (ϵ) = \frac{1}{V} \sum_{\vec{k}} δ (ϵ - ϵ_{\vec{k}}) = \frac{1}{L^{3}} \frac{1}{{(\frac{2 π}{L})}^{D}} \int d \vec{k} δ (ϵ - ϵ_{\vec{k}}) = \frac{1}{(2 π)^{D}} \int d \vec{k} δ (ϵ - ϵ_{\vec{k}}) = {\begin{cases} 4 π \int d k k^{2} & D = 3 \\ 2 π \int d k k^{1} & D = 2 \\ 2 \int d k k^{0} & D = 1 \end{cases}$ . Then, $k \to ϵ$ . $d ϵ = | \frac{d ϵ}{d k} | d k$ .

For phonons, $g (k) = 2$ , so it is typically not written.

Blackbody Radiation

$ω = c k$ . $c = ν \cdot λ = \frac{ω}{2 π} \frac{2 π}{k}$ . $ϵ_{p h} = ℏ c k = ℏ ω$ .

Consider a box with a small hole in it. Put a photonic gas (Electromagnetic Radiation) in the box at a temperature $T$ .

$g (ω) d ω = 2 \frac{V}{(2 π)^{3}} 4 π k^{2} \frac{d k}{d ω} d ω$ Note the leading two comes from $g (k) = 2$ for the photonic gas. Then, $k^{2} = \frac{ω^{2}}{c^{2}}$ . $\frac{d k}{d ω} = \frac{1}{c}$ .

Then, $g (ω) d ω = \frac{V ω^{2}}{π c^{3}} d ω$ .

The energy density is then, $U (ω) d ω = V u (ω) d ω = ϵ (ω) g (ω) n_{p h} (ω, T) d ω$ . $n_{p h} (ω, T) = n_{B} (μ = 0)$ .

$U (ω) d ω = V u (ω) d ω = \frac{ℏ ω g (ω)}{\exp (β ℏ ω) - 1} d ω = \frac{V ℏ ω^{3}}{π^{2} c^{3}} \frac{1}{\exp (β ℏ ω) - 1} d ω$ . So, $u (ω) = \frac{ℏ ω^{3}}{π^{2} c^{3}} \frac{1}{\exp (β ℏ ω) - 1}$ .

Aside

Remember that $n_{F} = \frac{1}{\exp (β (ϵ (k) - μ)) - 1}$ .

Lecture 2

Consider that we have energy levels $ϵ_{i}$ for each particle. So, classically, $⟨ ϵ ⟩ = \sum p_{n} ϵ_{n}$ and $⟨ E ⟩ = N ⟨ ϵ ⟩ = \sum N p_{n} ϵ_{n} = \sum n ϵ_{n}$ . Quantum mechanically, $⟨ ϵ ⟩ = \sum_{k} n^{F D / B E} (ϵ_{k}, μ, T) ϵ_{k}$ .

Remember for fermions, if there is a particle in $ϵ_{1}$ then there are no other particles in $ϵ_{1}$ .

Other Boson Systems

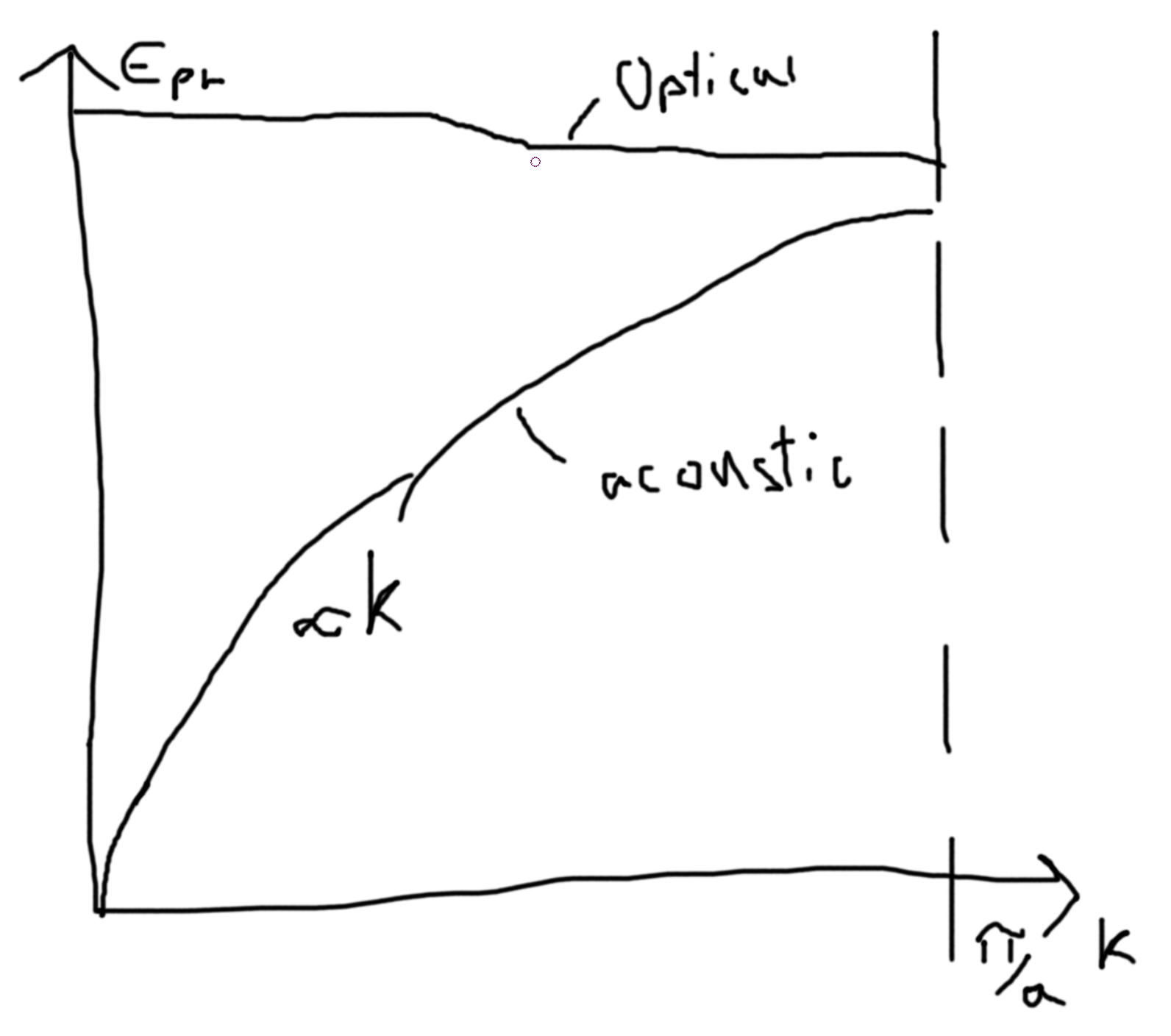
Lattice vibrations in solids (phonons). 20230130110456-statistical_mechanics.org_20230407_111806.png

2 Models:

Einstein model $ϵ_{p h} = ℏ ω_{0} = c o n s t$ . We did this microcanonically in HW 641. $U (T) = 3 N_{A} \frac{ℏ ω_{0}}{\exp (\frac{ℏ ω}{k T}) - 1}$ . Low temp, $C_{V} \propto \exp (- \frac{ℏ ω_{0}}{k T})$ .
Debye Model $ω (k) = v_{S} k$ . Where $v_{S} = \frac{\partial ω}{\partial k}$ is the speed of sound. That makes the acoustic curve linear. Has to fix $N$ for the 3N modes. Find $ω_{m a x}$ from fixing $N$ phonons which is $3 N_{A}$ times number of atoms. For low temperature, $C_{V} (T) \propto T^{3}$ which is correct and Einstein’s model has the wrong prediction.

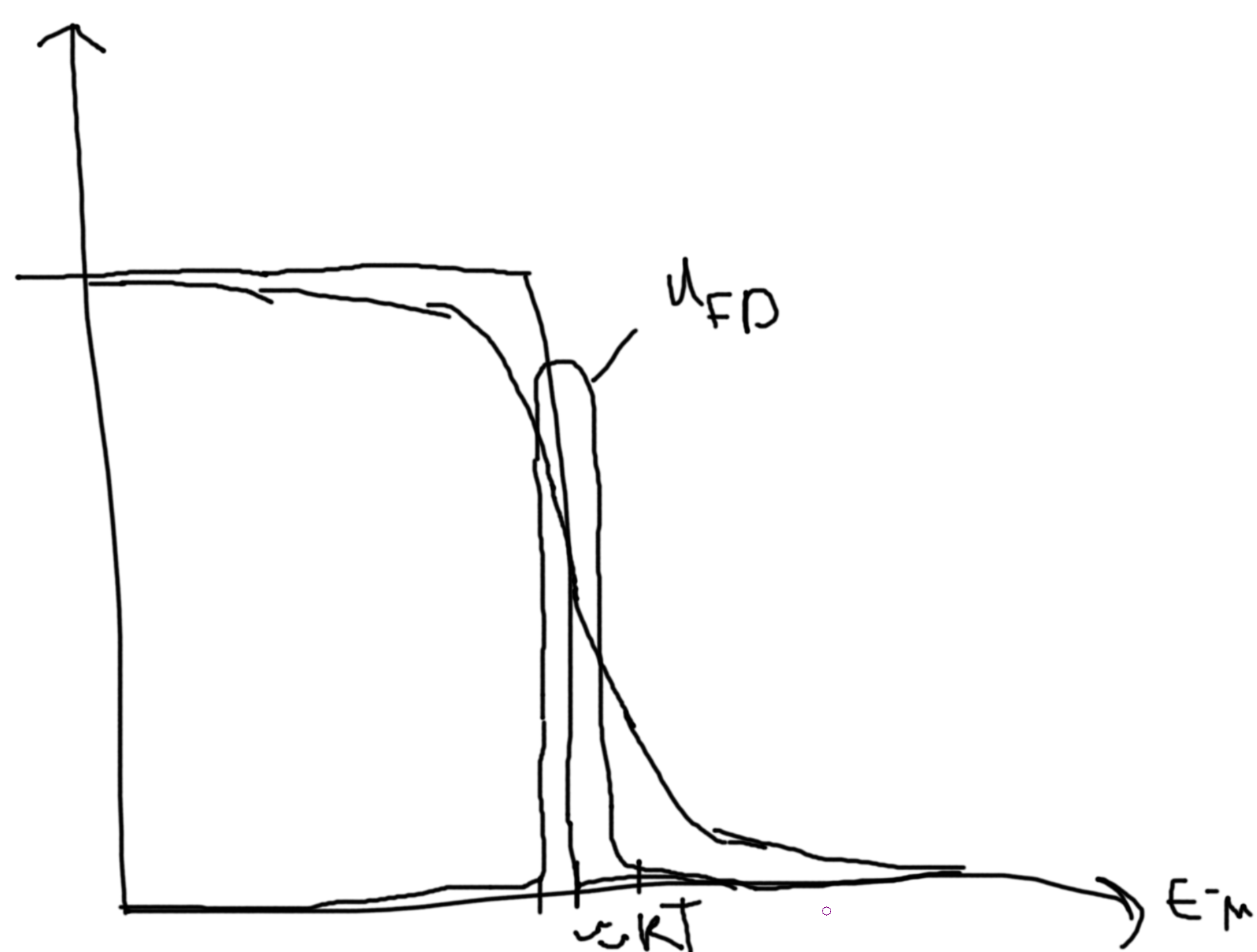
Fermions

They obey Fermi-Dirac statistics.

$u_{F D} = \frac{1}{\exp (β (ϵ - μ)) + 1}; ϵ (k) = \frac{ℏ^{2} k^{2}}{2 m}$ . The density of states is then $g (ϵ) = \frac{1}{4 π^{2}} {(\frac{2 m}{ℏ^{2}})}^{3 / 2} \sqrt{ϵ}$ and $N = \int d ϵ u_{F D} (ϵ, μ, T) g (ϵ)$ .

Can’t solve in general, so we do it in 3 stages.

$T = 0$ (HW)
High $T$
Low $T$

For $T = 0$ . $u_{F D} = Θ (x)$ . $μ (T = 0) = ϵ_{F}$ . So we want $N = \int d ϵ Θ (μ (T = 0) - ϵ) V g (ϵ)$ . Then, $= \int_{0}^{ϵ_{F}} d ϵ (2) \frac{V}{4 π^{2}} {(\frac{2 m}{ℏ^{2}})}^{3 / 2} \sqrt{ϵ}$ . The two comes from spin 1/2. $n = \frac{N}{V} = \dots = \frac{1}{3 π^{2}} {(\frac{2 m}{ℏ^{2}})}^{3 / 2} ϵ_{F}^{3 / 2}$ . Thus, $ϵ_{F} = {(3 π n)}^{2 / 3} \frac{ℏ^{2}}{2 m}$ .

$N = \sum_{\vec{k}, σ} n_{F D} (T = 0, ϵ_{k}) = 2 \frac{V}{(2 π)^{3}} \int d k Θ (ϵ_{F} - ϵ)$ . $Θ (ϵ_{F} - ϵ) \to Θ (k_{F} - k)$ from $ϵ_{F} = \frac{ℏ^{2} k_{F}^{2}}{2 m}$ .

For high $T$ . Recall for an ideal gas: $F = N k T (\ln \frac{N}{V} λ^{3} - 1)$ eq [6.24]. $μ = {\frac{\partial F}{\partial N} |}_{T, V} = \dots = k T \ln (n λ^{3})$ . Then, for our case, $u_{F D} = \frac{1}{\exp (β (ϵ - μ)) + 1} \to_{μ < ϵ} \exp (- β (ϵ - μ))$ . So, $N / V = n = \int d ϵ \exp (- β (ϵ - μ)) 2 \frac{1}{4 π^{2}} {(\frac{2 m}{ℏ^{2}})}^{3 / 2} \sqrt{ϵ} = \dots = {(\frac{2 m}{ℏ})}^{3 / 2} \sqrt{π} (k T)^{3} \exp (μ / k T)$ . Solving this for $μ$ gives you $μ = k T \ln (n λ^{3})$ .

For low $T$ . So, $k T ≪ ϵ_{F}$ . The typical scale of $ϵ_{F}$ is roughly $4 - 5 e V$ . Note that $1 e V \sim 10000 K$ .

$\frac{ϕ}{V} = 2 (- b (μ) - \frac{π^{2}}{6} g (μ) (k T)^{2} + O (k T)^{4})$ , $b (μ) = \int_{- \infty}^{μ} d ϵ a (ϵ)$ and $a (ϵ) = \int_{- \infty}^{ϵ} d \tilde{ϵ} g (\tilde{ϵ})$ .

$Z_{G} = \prod_{k} Z_{G_{k}} = \prod_{k} (1 + \exp (- \frac{ϵ_{K} - μ}{k T}))$ , $ϕ = - k T \ln Z_{G} = - k T \sum_{n} \ln (1 + \exp (- \frac{ϵ_{n} - μ}{k T})) \to - k T V \int_{- \infty}^{\infty} d ϵ g (ϵ) \ln (1 + \exp (- \frac{- ϵ - μ (T)}{k T}))$ Invert $N = N (μ (T))$ to get $μ (T)$ . Get $N$ from $N = - {(\frac{\partial ϕ}{\partial μ})}_{V, T} = 2 (\frac{\partial b}{\partial μ} + \frac{π^{2}}{6} \frac{\partial g (ϵ)}{\partial ϵ} |_{μ} (k T)^{2})$ . $\frac{\partial b}{\partial μ} = a (μ) \approx a (ϵ_{F}) + (μ - ϵ_{F}) {(\frac{\partial a}{\partial ϵ})}_{ϵ_{F}} = \frac{N}{2} + (μ - ϵ_{F}) g (ϵ_{F})$ . $N = 2 (\frac{1}{2} N + (μ - ϵ_{F}) g (ϵ_{F}) + \frac{π^{2}}{6} {(\frac{\partial g (ϵ)}{\partial ϵ})}_{ϵ_{F}} (k T)^{2})$ . Thus, the second and third term must equal zero. So, $μ (T) = ϵ_{F} - \frac{π^{2}}{6} \frac{1}{g (ϵ_{F})} {(\frac{\partial g}{\partial ϵ})}_{ϵ_{F}} (k T)^{2}$

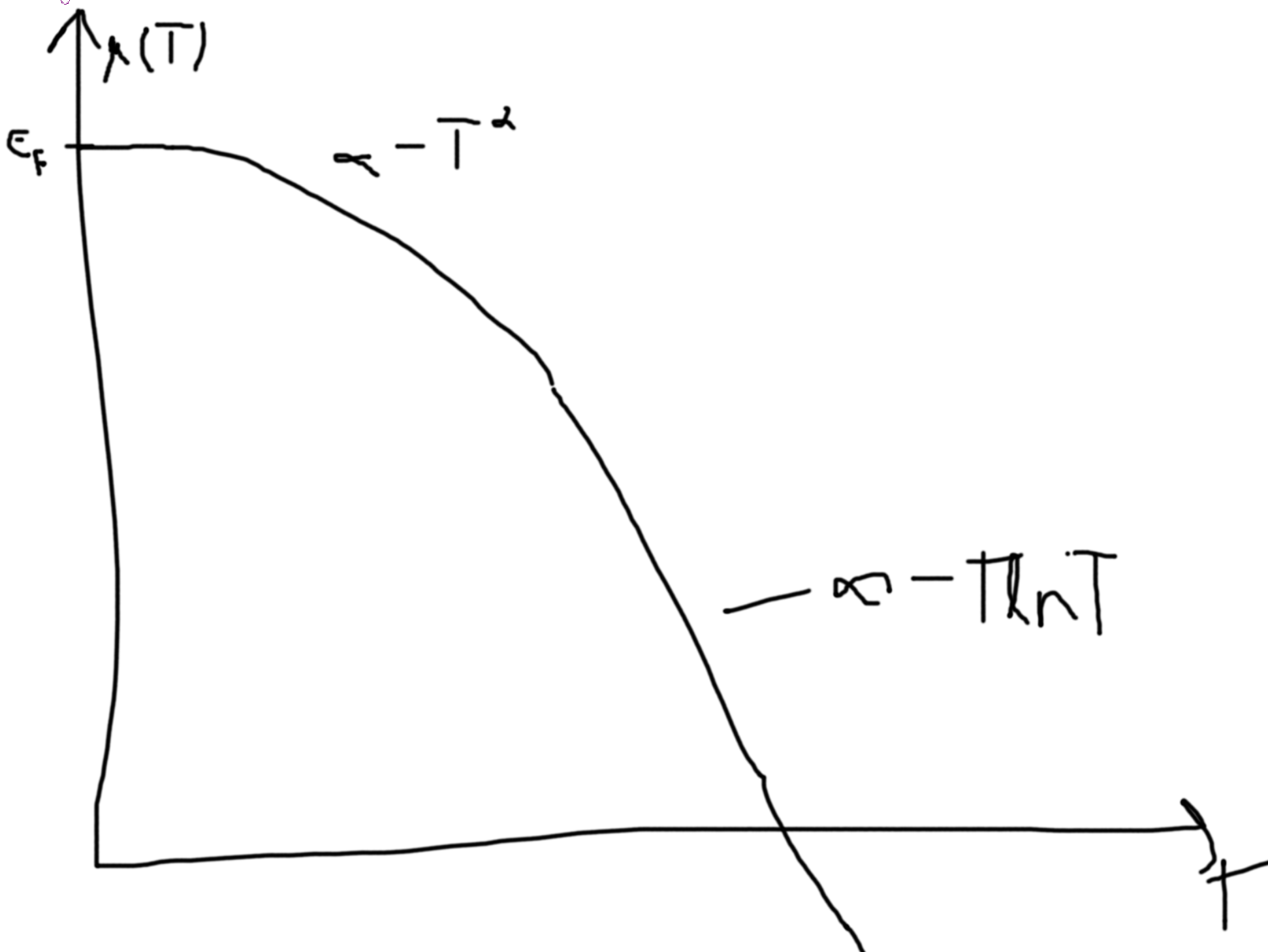
20230130110456-statistical_mechanics.org_20230407_115429.png .

20230130110456-statistical_mechanics.org_20230407_114709.png

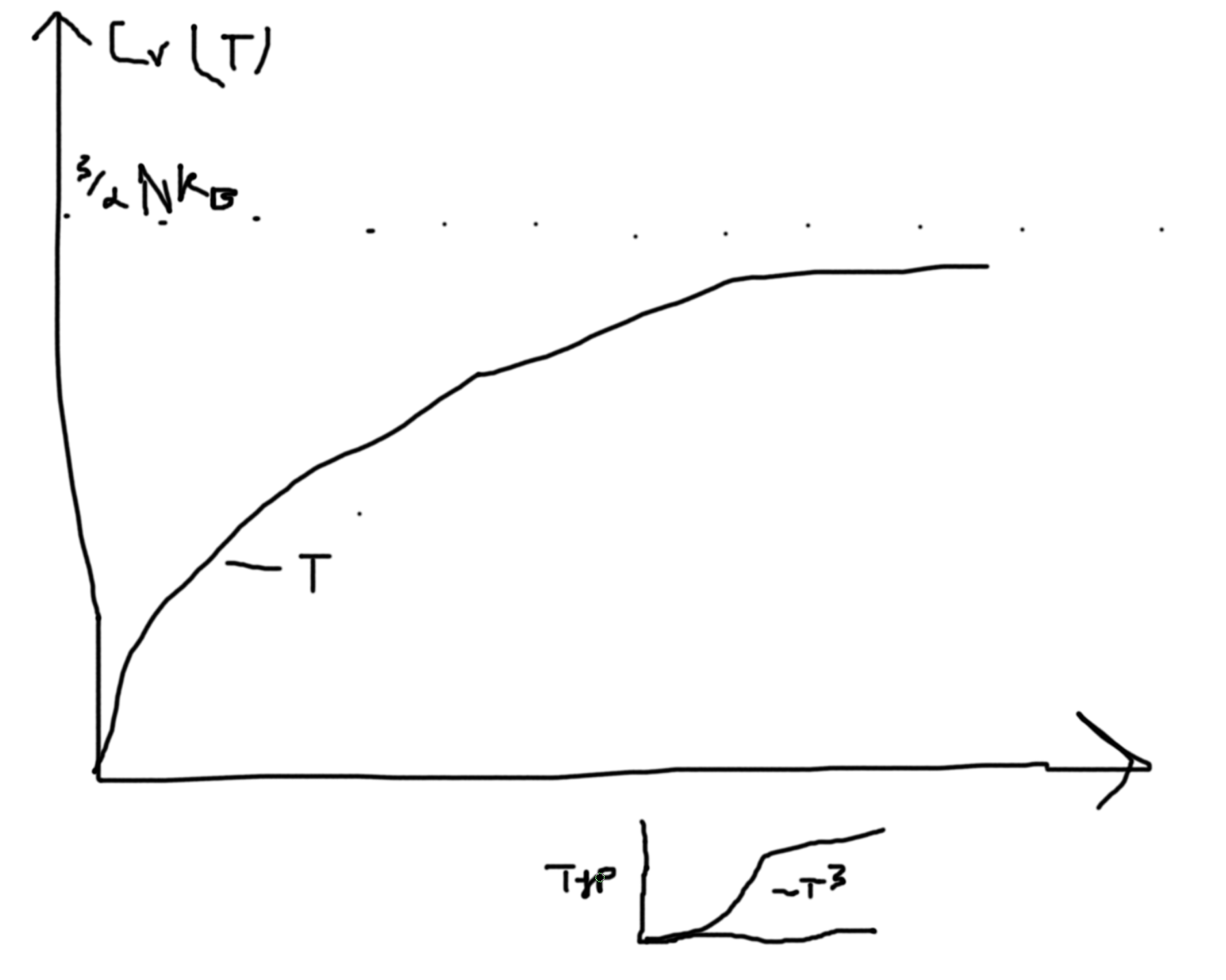
20230130110456-statistical_mechanics.org_20230407_115632.png

Electrons are fermions with spin 1/2. $2 g_{F} = g_{e -}$